|
Infinite Series
An infinite series is an expression such as: 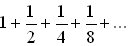 where
the dots imply that an infinite number of terms will be added. where
the dots imply that an infinite number of terms will be added.
|
|
To find the sum of an infinite series, we exam the partial sums. The sum of the series will be the
limit of the partial sums (that is, the number that the
partial sums are approaching, if, in fact, they do approach
a number). |
If the limit
exists, the series is said to converge.
If the limit does not exist the series is said to diverge. |
 |
The sum of an infinite
series is the limit of the partial sums.
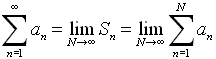
On the graphing calculator:
 |